The Manhole
Solution

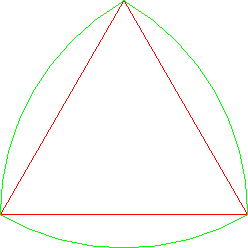
Surprisingly, the circle is not the only shape with constant diameter. For one example, consider the "inflated triangle". Start with an equilateral triangle, and replace each side by the arc of a circle whose center is the opposite vertex. This shape, known as the Reuleaux triangle, has constant diameter.

It may not be obvious that this guarantees that the manhole cover won't fall into the manhole. But it does. What you have to do is imagine someone push the manhole cover through the manhole while you watch from the side. At all times, the manhole cover can be marked by a single straight line that indicates the part that is currently in the "mouth" of the hole. This line can never be longer than the diameter of the hole, which we will call D.

If the manhole cover can pass through the hole, we have to guarantee that we can move a line across the manhole cover in such a way that the line is always strictly less than D. This is the same as imagining that you place both fingers at one spot on the manhole cover, move one to the left and one to the right, in such a way that they are always less than D units apart until they meet again. But this can't happen.

The reason it can't happen is that there are three "corner points", each of which is D units away from all the points on its opposite side. You can show that there is no way to push the manhole cover through the hole without, at some point, having one of the corner points opposing a point of its opposite side. But then the part of the manhole cover we are pushing through is D units wide, and we said we'd always be working with less than D units.

This puzzle was inspired by a misleading statement attributed to Danica McKellar of The Wonder Years:

"...For instance, one of them is the question: Why are manhole covers round? Well, if you had a square shape, for instance, you could pick up the lid, tilt it, and it would fall through. A circle is the only shape that has a constant diameter. And that's an interesting thing. Then to realize that's the definition of a circle, actually."

1. Martin Gardner,
   The Unexpected Hanging,
   Chapter Eighteen: Curves of Constant Width,
   Simon and Shuster, 1968.
2. Deanna Haunsperger and Stephen Kennedy,
   The Number Years,
   Math Horizons,
   April 2001, pages 5-7.
3. http://www.figurethis.org, "Figure This", Math Challenges for the Family.

Thanks to Jim Fink for pointing out and discussing this puzzle with me.